The most striking features of crystals are their symmetric, polyhedral forms. Federov said that crystals “flash-forth their symmetry”, a sharp statement about sharp, highly reflecting surfaces of well-formed crystals. Not uncommonly the interactions of crystals with light that scientists would expect, given apparent crystal shape or symmetry, are not those seen in practice. They deviations between expectation and measurement, unanticipated linear birefringences, are subtle indications of the mysterious properties of growth mechanisms, how and why the ions or molecules got to where they are going in the first place.

Unlike easily measured linear birefringence, circular birefringence or optical rotation, the difference in refractivity for counterpropagating circularly polarized waves, is usually a tiny perturbation to the state of polarization of a beam propagating through a crystal. A low symmetry crystal may be burdened with anisotropies that render the measurement of optically activity in crystals extremely difficult. This problem has lingered as a since the discovery of optical rotatory dispersion more than 200 years ago. Our group has built polarimetric devices for making measurements of this kind that affect the determination of chiroptical anisotropies more rapidly than has been accomplished previously. Such measurements are essential for determining the complex magnetoelectric tensor that determines circular birefringence and circular dichroism.

Virtually every civilization has developed technologies for dyeing textiles. On the other hand, we do not often practice the dyeing of crystals. The reason for this distinction is plain: fibrous materials such as wool or cotton, have large surface area-to-mass ratios compared with polyhedral crystals. However, the surface area of a growing crystal, the sum of the surface areas after the accretion of each new ionic or molecular layer, is enormous. As a matter of fact, one can find in the descriptive crystallographic literature of the last 150 years examples of simple transparent crystals stained by dyes during growth from solution. When dyes express different affinities for the various facets of a growing crystal, they produce striking patterns of color that are determined by the host crystal’s symmetry.Such artificially colored transparent crystals inform crystal growth mechanisms and can form the basis of new optically responsive materials.

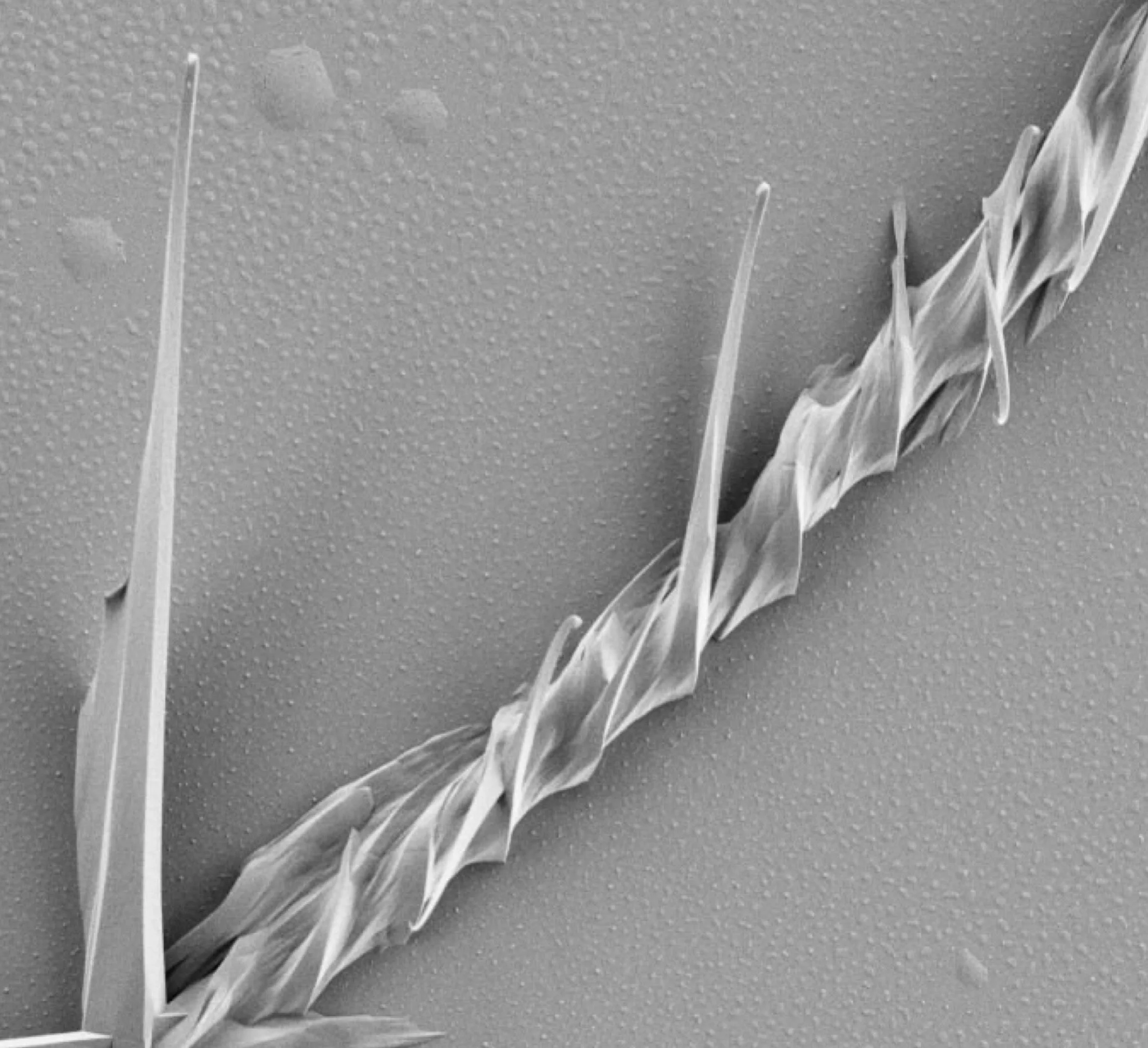
In 1929, Ferdinand Bernauer (1890–1945) concluded that about one quarter of simple molecular crystals can be made to grow as mesoscale helices. Bernauer’s judgment was based on analyses of the optical properties and morphologies of more than 400 melt-grown crystals of substances that he was able to obtain from colleagues throughout Germany. His far-reaching judgment about crystal morphology, of which recent monographs on crystal growth say not a word, has changed our view of crystals and crystallography. After some study, we now know Bernauer’s claim to be true. But, how and why? By what mechanism can a large proportion of molecular crystals spontaneously form helicoidal structures.

The World Health Organization’s 1955 assault on malaria carrying mosquitoes using the insecticide DDT almost succeeded in wiping out the most pernicious infectious disease in human history. Here, we consider a counterfactual science scenario: What if DDT had been a little faster, thereby combatting the inevitable development of resistance to the compound, a biological response ultimately doomed worldwide malaria eradication. A faster-acting difluoro DDT congener, DFDT, was developed in Germany during World War II but its purported superior performance against insects and reduced toxicity to mammals compared to DDT were dismissed by Allied military inspectors in 1945. DFDT was never again manufactured. We aimed to establish whether a broad-spectrum insecticide, DFDT, is hiding in plain view. Here, we show the efficacy of amorphous and crystalline forms of DFDT and a mono-fluorinated chiral congener, MFDT, all previously unknown, against Drosophila as well as Anopheles and Aedes mosquitoes. We compared the lethality of two crystalline polymorphs of DFDT, four crystals of chiral MFDT (racemic and resolved), as well as the respective persistent amorphous solids. Our results demonstrate that crystalline DFDT and MFDT kill faster than DDT, and their amorphous forms were even faster, which is essential for mitigating vector resistance. We also demonstrate an unambiguous inverse relationship between lethality and thermodynamic stability of solid forms, which suggests a crystal engineering strategy for improving the efficacy of known insecticides by tailoring crystal form. We anticipate that the fluorinated congeners, properly evaluated, may be effective in the battle against insect-borne diseases.

Optical rotations and rotatory strengths are calculated for planar, conjugated hydrocarbons with the aim of determining to what extent the sum-over-π→π* rotatory strengths or even more restricted sets of wave functions are sufficient to account for non-resonant optical activity, as well as to what extent qualitative molecular orbital theory can be used to interpret chiroptical structure-property relations. It is shown that by restricting our analyses to planar π-systems, an intuitive understanding of the vexing property of optical activity is forthcoming for the following reasons: Wave functions under the Hückel approximations are simply determined and graphically computed to yield transition dipole and quadrupole moments; the forms of the gyration tensors are given by symmetry with simple representation surfaces whose orientations are completely or partly determined by symmetry; transition electric dipole and magnetic dipole moments have fixed, orthogonal dispositions relative to one another, and the most optically active directions are found at their bisectors. Throughout, the emphasis is on reckoning long wavelength optical rotation using simple models that are part of organic chemistry pedagogy.